Well, the only plausible explanation (see Trident) for the high drag of the YB49 is depending on the t/c ratio (which is directly linked to the frontal area) and so far neither you nor Henning came up with another explanation. You can try to focus on other planes with different problems, but this thread started (by being seperated) with the comparison of the B35/49 with the B47.
You are using an out of date browser. It may not display this or other websites correctly.
You should upgrade or use an alternative browser.
You should upgrade or use an alternative browser.
Frontal area vs. drag vs. wetted area vs
- Thread starter Nicknick
- Start date
- Status
- Not open for further replies.
Hi Nicknick,
It might be worth looking at your post number #1 again:
That's a an perfectly general statement not limited to any particular aircraft, on which I expressed my doubts right in post #2:
As everyone has been telling you, the YB-49 vs. B-47 comparison is a cherry-picked example. It might coincedentally fit your claim, but that doesn't prove your initial claim.
Here's a quote from Kelly Johnson himself (via https://www.nurflugel.com/Nurflugel/Northrop/Northrop_address/body_northrop_address.html ):
As he's discussing the same topic you like to talk about, maybe you would like to explain why you believe Johnson's was wrong about the parasitic drag for comparative airplanes of the same span and gross weight.
Regards,
Henning (HoHun)
You can try to focus on other planes with different problems, but this thread started (by being seperated) with the comparison of the B35/49 with the B47.
It might be worth looking at your post number #1 again:
Flying wings offer very good glide numbers and a high lift (at low speeds) but they have a large frontal area (=> high parasitic drag).
That's a an perfectly general statement not limited to any particular aircraft, on which I expressed my doubts right in post #2:
Hi Nicknick,
Hm, what's the source for that opinion? I'm not sure I follow ...Flying wings with jet engines was the coolest stuff in those times, but this combination was neither suitable for maximum range or speed. Flying wings offer very good glide numbers and a high lift (at low speeds) but they have a large frontal area (=> high parasitic drag).
Regards,
Henning (HoHun)
As everyone has been telling you, the YB-49 vs. B-47 comparison is a cherry-picked example. It might coincedentally fit your claim, but that doesn't prove your initial claim.
Here's a quote from Kelly Johnson himself (via https://www.nurflugel.com/Nurflugel/Northrop/Northrop_address/body_northrop_address.html ):
The ratio of the minimum parasite drag coefficient (CDmin) for all-wing airplanes to that for conventional types is approximately 1:2. Minimum drag coefficients for a number of large bomber and transport aircraft such as the B-29, B-24, C~4 and others average approximately .023. The minimum drag coefficients for several all-wing types have been measured both in model and full-scale configurations and vary from less than .010 to about .0113, which is the figure for the XB-35 including armament protuberances, drive shaft housings, rudimentary nacelle for gun emplacements, and so on.
The ratio of maximum trimmed lift coefficient (Clmax) for all-wing to conventional types is approximately 1.5:2.3. The latter figure is typical for a number of the large airplanes of conventional arrangement previously mentioned. The former is readily attainable in a configuration such as that of the XB-35 and may be subject to considerable improvement through the use of several types of high lift devices yet to be proved.
For comparative airplanes of the same span and gross weight the selection of the required wing area will depend either on flight conditions, including takeoff without flaps, or landing conditions. If the flight conditions govern, the ratio of required wing areas of all-wing to conventional aircraft will be 1:1 because the two wings are equally effective except under conditions of maximum lift. If landing conditions govern, the ratio will be 21 3:1, assuming the same landing speed in each case. If takeoff with partial flap deflection governs, the ratio will be somewhere between the above two figures. In large all-wing bombers and transports, and a growing extent in conventional long-range transports as well, the ratio of gross weight at takeoff to landing weight will approach 2:1. Therefore flight conditions are likely to govern the selection of wing area more than landing conditions. In the following calculations both extremes are used as indicative of the range of advantage to be gained by the use of the all-wing configuration. Referring to Fig. 1, it may be seen from equation (1) that the total minimum parasite drag of the all-wing airplane in terms of the conventional airplane will vary from 50 percent if flight conditions govern, to 77 percent if landing conditions govern. In this equation (Dp)a and (Dp)c represent the parasite drags of all-wing and conventional airplanes while Sa and Sc represent the respective wing areas.

As he's discussing the same topic you like to talk about, maybe you would like to explain why you believe Johnson's was wrong about the parasitic drag for comparative airplanes of the same span and gross weight.
Regards,
Henning (HoHun)
- Joined
- 8 March 2009
- Messages
- 1,042
- Reaction score
- 1,220
Well, the only plausible explanation (see Trident) for the high drag of the YB49 is depending on the t/c ratio (which is directly linked to the frontal area) and so far neither you nor Henning came up with another explanation. You can try to focus on other planes with different problems, but this thread started (by being seperated) with the comparison of the B35/49 with the B47.
Simple question. There are two wings;
Wing A - 1ft chord 20% t/c
Wing B - 2ft chord 8% t/c
Which has higher drag?
djfawcett
With Enough Power, Anything Will Fly
- Joined
- 16 December 2012
- Messages
- 252
- Reaction score
- 357
Your simple mathematics is incorrect. A would be in square units. T is a unitless percent. B is in linear units. T X B doesn't equal anything squared. Always remember to include units. First thing learned in physics.you cant have one without the other (on a flying wing). If a flying wing has a low wetted area but a high wingthinkness it implies that it has a large frontal and high t/c ratio, the mathematics are quite simple. The frontal area geometry of the YB49 can be well describt as two triangles, with a maximum thikness of about two meters in the middle. So the frontal area is AFrontal = 1/2 * t max * b
with (might be not the best letters)
t = maximum thikness
b= wingspan
A = frontal area
This is no oversimplification but simple mathematics. You can try to blure the facts by overcomplivication, but this is not what engineering is about.T
I wrote t = maximum thickness = 2m, so according to my own definition it has a dimension (As well as the wingspan which has 52 m) As said, I’m not familiar which letters are used by Anglo-Saxon aerodynamic equations, therefore I defined my letters, you sould have read this before starting commenting.Your simple mathematics is incorrect. A would be in square units. T is a unitless percent. B is in linear units. T X B doesn't equal anything squared. Always remember to include units. First thing learned in physics.you cant have one without the other (on a flying wing). If a flying wing has a low wetted area but a high wingthinkness it implies that it has a large frontal and high t/c ratio, the mathematics are quite simple. The frontal area geometry of the YB49 can be well describt as two triangles, with a maximum thikness of about two meters in the middle. So the frontal area is AFrontal = 1/2 * t max * b
with (might be not the best letters)
t = maximum thikness
b= wingspan
A = frontal area
This is no oversimplification but simple mathematics. You can try to blure the facts by overcomplivication, but this is not what engineering is about.T
I don’t see any relevance in your posting
Hi Nicknick,
You can try to focus on other planes with different problems, but this thread started (by being seperated) with the comparison of the B35/49 with the B47.
It might be worth looking at your post number #1 again:
Flying wings offer very good glide numbers and a high lift (at low speeds) but they have a large frontal area (=> high parasitic drag).
That's a an perfectly general statement not limited to any particular aircraft, on which I expressed my doubts right in post #2:
Hi Nicknick,
Hm, what's the source for that opinion? I'm not sure I follow ...Flying wings with jet engines was the coolest stuff in those times, but this combination was neither suitable for maximum range or speed. Flying wings offer very good glide numbers and a high lift (at low speeds) but they have a large frontal area (=> high parasitic drag).
Regards,
Henning (HoHun)
As everyone has been telling you, the YB-49 vs. B-47 comparison is a cherry-picked example. It might coincedentally fit your claim, but that doesn't prove your initial claim.
Here's a quote from Kelly Johnson himself (via https://www.nurflugel.com/Nurflugel/Northrop/Northrop_address/body_northrop_address.html ):
The ratio of the minimum parasite drag coefficient (CDmin) for all-wing airplanes to that for conventional types is approximately 1:2. Minimum drag coefficients for a number of large bomber and transport aircraft such as the B-29, B-24, C~4 and others average approximately .023. The minimum drag coefficients for several all-wing types have been measured both in model and full-scale configurations and vary from less than .010 to about .0113, which is the figure for the XB-35 including armament protuberances, drive shaft housings, rudimentary nacelle for gun emplacements, and so on.
The ratio of maximum trimmed lift coefficient (Clmax) for all-wing to conventional types is approximately 1.5:2.3. The latter figure is typical for a number of the large airplanes of conventional arrangement previously mentioned. The former is readily attainable in a configuration such as that of the XB-35 and may be subject to considerable improvement through the use of several types of high lift devices yet to be proved.
For comparative airplanes of the same span and gross weight the selection of the required wing area will depend either on flight conditions, including takeoff without flaps, or landing conditions. If the flight conditions govern, the ratio of required wing areas of all-wing to conventional aircraft will be 1:1 because the two wings are equally effective except under conditions of maximum lift. If landing conditions govern, the ratio will be 21 3:1, assuming the same landing speed in each case. If takeoff with partial flap deflection governs, the ratio will be somewhere between the above two figures. In large all-wing bombers and transports, and a growing extent in conventional long-range transports as well, the ratio of gross weight at takeoff to landing weight will approach 2:1. Therefore flight conditions are likely to govern the selection of wing area more than landing conditions. In the following calculations both extremes are used as indicative of the range of advantage to be gained by the use of the all-wing configuration. Referring to Fig. 1, it may be seen from equation (1) that the total minimum parasite drag of the all-wing airplane in terms of the conventional airplane will vary from 50 percent if flight conditions govern, to 77 percent if landing conditions govern. In this equation (Dp)a and (Dp)c represent the parasite drags of all-wing and conventional airplanes while Sa and Sc represent the respective wing areas.
View attachment 674309
As he's discussing the same topic you like to talk about, maybe you would like to explain why you believe Johnson's was wrong about the parasitic drag for comparative airplanes of the same span and gross weight.
Regards,
Henning (HoHun)
I posted a trustworthy quotation which shows, that the parasitic drag of the B49 was more than twice than that of the B47.
I was comparing two planes (same kind of job, about the same jet trust and weight) with similar wetted areas, whereas Johnson was comparing planes with similar wingspan. This is not the same!
As we know, flying wings tend to need higher lift designs because they lack flaps. Also they tend to have a high wing thickness (not all, see B2, but most) to enable enough internal height and volume. Usually, if you increase the lift by making the wings thicker (without changing the wingspan) you increase drag, even if the L/D ratio would remain constant. The B49 had a much higher c/t ratio which resulted in having more lift and drag than the B47.
To meet Mr Johnsons boundary conditions, the wings of the B49 would have had to be much thinner and with smaller span In this case, I would totally expect, Mr Johnson to be right, the B49(small) would have very likely had a lower drag than the B47, but it would have been an entirely different airplane.
Hi Nicknick,
You posted one set of graphs with numbers, but not the text explaining their significance. That's nice for starters, but parasitic drag depends on Mach number at operational speeds of the types considered, and we don't know if that's included in the numbers or not. Does the wetted area include the interior surfaces of the wing slots and the extensive intake ducts of the YB-49, and if so, for which flight and intake velocity? So, you get some brownie points for trying, but you haven't actually shown anything, though I certainly found the graphs interesting to look at.
Exactly. You were making an absolute statement addressing not any specific type, but flying wings in general. Then you proceeded to pick an aircraft originally designed for a completely different working point to prove your general point. No poster here has accepted that as conclusive evidence so far, yet you keep bringing it back up again in nearly every post you make. Save the ink, your example is worthless.
Just to illustrate the difference between the two planes, based on their SAC sheets:
- The YB-49 has a design weight of 214000 lbs.
- The B-47A has a design weight of 125000 lbs.
- The YB-49 with a 16000 lbs bomb load has a combat range of 2520 nm with a total mission time of 7.00 hours.
- The B-47A with a 10000 lbs bomb load has a combat range of 2634 nm with a total mission time of 6.21 hours.
So, apples and oranges.
Is this supposed to be Nicknick t or conventional t? If you'd invested a bit more mental effort, you'd have found that aerodynamicists universally use the t/c ratio. If you were in fact trying to address the chord-to-thickness ratio, the B-49's would actually be lower. Guess the crescent wrench came done on the fingernail this time! :-D
Regards,
Henning (HoHun)
I posted a trustworthy quotation which shows, that the parasitic drag of the B49 was more than twice than that of the B47.
You posted one set of graphs with numbers, but not the text explaining their significance. That's nice for starters, but parasitic drag depends on Mach number at operational speeds of the types considered, and we don't know if that's included in the numbers or not. Does the wetted area include the interior surfaces of the wing slots and the extensive intake ducts of the YB-49, and if so, for which flight and intake velocity? So, you get some brownie points for trying, but you haven't actually shown anything, though I certainly found the graphs interesting to look at.
I was comparing two planes (same kind of job, about the same jet trust and weight) with similar wetted areas, whereas Johnson was comparing planes with similar wingspan. This is not the same!
Exactly. You were making an absolute statement addressing not any specific type, but flying wings in general. Then you proceeded to pick an aircraft originally designed for a completely different working point to prove your general point. No poster here has accepted that as conclusive evidence so far, yet you keep bringing it back up again in nearly every post you make. Save the ink, your example is worthless.
Just to illustrate the difference between the two planes, based on their SAC sheets:
- The YB-49 has a design weight of 214000 lbs.
- The B-47A has a design weight of 125000 lbs.
- The YB-49 with a 16000 lbs bomb load has a combat range of 2520 nm with a total mission time of 7.00 hours.
- The B-47A with a 10000 lbs bomb load has a combat range of 2634 nm with a total mission time of 6.21 hours.
So, apples and oranges.
The B49 had a much higher c/t ratio which resulted in having more lift and drag than the B47.
Is this supposed to be Nicknick t or conventional t? If you'd invested a bit more mental effort, you'd have found that aerodynamicists universally use the t/c ratio. If you were in fact trying to address the chord-to-thickness ratio, the B-49's would actually be lower. Guess the crescent wrench came done on the fingernail this time! :-D
Regards,
Henning (HoHun)
As always, you tend to not believe my sources if they didn’t fit to your ideas. My link is from a book written by two Proffessors for aerospace engineering of the University of Kansas, so it is very reasonable to believe, that they knew what they were doing and that they compared airplanes by similar conditions in their charts.
You number for the weight of the YB49 appear much to high, all I could find shows that the empty weight was about 40 tons and the maximum starting weight was about 88 tons
The empty weight of the B47 was 36 tons, the maximum take off weight was 100 tons, so both planes are comparable in weight and the B47 having not only less parasitic drag, but also more starting weight.
So as we see, if you choose the correct numbers, both planes can be compared very well. Choosing two planes with similar properties and the same wetted area is totally legitimate, it you want to judge whether the wetted area is the only impotant factor for drag or not, and if wing thikness or frontal area play an important role. As we see, they do, and you can try hard to confuse this fact by bringing different examples or wrong numbers, but it doesn’t work.
PS: I don’t care what you write about wrenches and I haven’t seen your video
You number for the weight of the YB49 appear much to high, all I could find shows that the empty weight was about 40 tons and the maximum starting weight was about 88 tons
The empty weight of the B47 was 36 tons, the maximum take off weight was 100 tons, so both planes are comparable in weight and the B47 having not only less parasitic drag, but also more starting weight.
So as we see, if you choose the correct numbers, both planes can be compared very well. Choosing two planes with similar properties and the same wetted area is totally legitimate, it you want to judge whether the wetted area is the only impotant factor for drag or not, and if wing thikness or frontal area play an important role. As we see, they do, and you can try hard to confuse this fact by bringing different examples or wrong numbers, but it doesn’t work.
PS: I don’t care what you write about wrenches and I haven’t seen your video
I found another source (http://www.dept.aoe.vt.edu/~mason/Mason_f/NorthropYB-49.pdf) about the YB49 with some more detailed information, including the aerofoils used in the YB49. This information also makes clear, that my information of maximum take off weight was correct.
The airfoils used (http://airfoiltools.com/airfoil/details?airfoil=naca653618-il) were indeed very thick, which comes to little surprise. For sufficient internal space and height, flying wings tend to use thick airfoils. As we see in the L/D chart, the wings lost efficiency when going from Mach 0.2 to Mach 0.8 but not dramatic, so with the difference in Mach numbers between the YB35 and YB49 being much smaller than 4, they would have worked nearly equal good for both airplanes. The high drag of the YB49 is not caused by a suddenly occurring flow separation at a certain Mach number, it is inherent in the design with the ultra-thick wing profile (= high frontal area).
In this paper we find also the simulated lift and drag ratio for the wing in cruise condition which are Cl: 0.118 and CD 0.00601 (Mach 0.5). With these values, the glide ratio of a perfect clean wing with the dimensions of the YB49 would be 19.6, of course, the real life airplane will be worse. I don’t know many glide ratios of jet planes, but to me, this glide ratio is not very convincing. Somewhere I found the information, that Jets can achieve about the same glide ratio (about 19 in cruise without the drag of the jets) and very efficient planes achieve even more than 20.
So, this paper fits very well to my analysis of the drawbacks from the YB49.
BTW. I don’t regard Discovery Channel qutations within the Wikipedia as very credible, but nevertheless I do quote it because it fits very well (retranslated into English from German):
"The conversion of the long-range XB-35 to jet engines essentially halved the aircraft's effective range and joins Boeing's new winged bomber, the B-47 Stratojet, in the medium-range bomber category. The B-47 was optimized for high-altitude, high-speed flight, and in an era where speed and altitude became the name of the game, the YB-49's thick profile could never be maximized for high-speed performance. In the same Discovery Channel documentary, former Air Force Flight Test Center historian Dr. James Young that while political gameplay and backroom trading certainly played a role in the plane's sinking, the Flying Wing program was ultimately canceled for sound technological reasons."
https://de.wikibrief.org/wiki/Northrop_YB-49
The airfoils used (http://airfoiltools.com/airfoil/details?airfoil=naca653618-il) were indeed very thick, which comes to little surprise. For sufficient internal space and height, flying wings tend to use thick airfoils. As we see in the L/D chart, the wings lost efficiency when going from Mach 0.2 to Mach 0.8 but not dramatic, so with the difference in Mach numbers between the YB35 and YB49 being much smaller than 4, they would have worked nearly equal good for both airplanes. The high drag of the YB49 is not caused by a suddenly occurring flow separation at a certain Mach number, it is inherent in the design with the ultra-thick wing profile (= high frontal area).
In this paper we find also the simulated lift and drag ratio for the wing in cruise condition which are Cl: 0.118 and CD 0.00601 (Mach 0.5). With these values, the glide ratio of a perfect clean wing with the dimensions of the YB49 would be 19.6, of course, the real life airplane will be worse. I don’t know many glide ratios of jet planes, but to me, this glide ratio is not very convincing. Somewhere I found the information, that Jets can achieve about the same glide ratio (about 19 in cruise without the drag of the jets) and very efficient planes achieve even more than 20.
So, this paper fits very well to my analysis of the drawbacks from the YB49.
BTW. I don’t regard Discovery Channel qutations within the Wikipedia as very credible, but nevertheless I do quote it because it fits very well (retranslated into English from German):
"The conversion of the long-range XB-35 to jet engines essentially halved the aircraft's effective range and joins Boeing's new winged bomber, the B-47 Stratojet, in the medium-range bomber category. The B-47 was optimized for high-altitude, high-speed flight, and in an era where speed and altitude became the name of the game, the YB-49's thick profile could never be maximized for high-speed performance. In the same Discovery Channel documentary, former Air Force Flight Test Center historian Dr. James Young that while political gameplay and backroom trading certainly played a role in the plane's sinking, the Flying Wing program was ultimately canceled for sound technological reasons."
https://de.wikibrief.org/wiki/Northrop_YB-49
Last edited:
- Joined
- 8 March 2009
- Messages
- 1,042
- Reaction score
- 1,220
The airfoils used (http://airfoiltools.com/airfoil/details?airfoil=naca653618-il) were indeed very thick, which comes to little surprise. For sufficient internal space and height, flying wings tend to use thick airfoils. As we see in the L/D chart, the wings lost efficiency when going from Mach 0.2 to Mach 0.8 but not dramatic, so with the difference in Mach numbers between the YB35 and YB49 being much smaller than 4, they would have worked nearly equal good for both airplanes. The high drag of the YB49 is not caused by a suddenly occurring flow separation at a certain Mach number, it is inherent in the design with the ultra-thick wing profile (= high frontal area).
I don't think you understand drag divergence, or much of anything else.
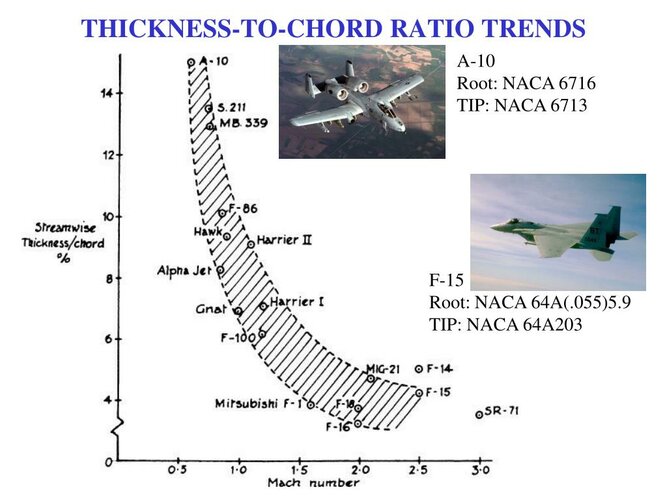
Again a simple question;
Wing A = 1ft chord 20% thick
Wing B = 2ft chord 8% thick
Which has more drag? Assume its a 65 series section.
Hi Nicknick,
I have clearly pointed out objective reasons why your (incomplete) source doesn't allow firm conclusions, and the material you have since posted nicely confirms my point that the drag coefficients are Mach-dependent. So, thanks for proving me right.
"All you could find" - well, you were not even trying.
The source I stated right before quoting the numbers are the SAC sheets, the link to which I provided earlier in this thread.
Also read my post again and note the term "design weight". That's a relevant figure because it correlates to the design working point for which the aircraft was optimized, which of course can diverge from the working work it's later operated at. You should have figured out by now that the YB-49 was operated far from the airframe's original design point ...
Note that I gave you an exact version of the B-47, which you failed to do. The B-47A's SAC sheet lists a maximum take-off weight of 157000 lbs, which is considerably less than the YB-47's basic mission take-off weight of 194000 lbs.
If you don't like the numbers, take it up with the Air Force ... it's hardly my fault you're too lazy to read the sources I clearly stated, and too ignorant to understand that "design weight" is not the same as "starting weight".
Regards,
Henning (HoHun)
As always, you tend to not believe my sources if they didn’t fit to your ideas. My link is from a book written by two Proffessors for aerospace engineering of the University of Kansas
I have clearly pointed out objective reasons why your (incomplete) source doesn't allow firm conclusions, and the material you have since posted nicely confirms my point that the drag coefficients are Mach-dependent. So, thanks for proving me right.
You number for the weight of the YB49 appear much to high, all I could find shows that the empty weight was about 40 tons and the maximum starting weight was about 88 tons
"All you could find" - well, you were not even trying.
The source I stated right before quoting the numbers are the SAC sheets, the link to which I provided earlier in this thread.
Also read my post again and note the term "design weight". That's a relevant figure because it correlates to the design working point for which the aircraft was optimized, which of course can diverge from the working work it's later operated at. You should have figured out by now that the YB-49 was operated far from the airframe's original design point ...
the B47 having not only less parasitic drag, but also more starting weight.
Note that I gave you an exact version of the B-47, which you failed to do. The B-47A's SAC sheet lists a maximum take-off weight of 157000 lbs, which is considerably less than the YB-47's basic mission take-off weight of 194000 lbs.
As we see, they do, and you can try hard to confuse this fact by bringing different examples or wrong numbers, but it doesn’t work.
If you don't like the numbers, take it up with the Air Force ... it's hardly my fault you're too lazy to read the sources I clearly stated, and too ignorant to understand that "design weight" is not the same as "starting weight".
Regards,
Henning (HoHun)
the maximum starting weight of the B47 is 100 tons, that more than that of the YB49. Despite the the starting weight of the B47 is higher, the key póint is, that the YB49 has more than twice the drag of the B47 with equal wetted area.
If you dont trust these proffessors, you could as well read that link: http://www.dept.aoe.vt.edu/~mason/Mason_f/NorthropYB-49.pdf which confirms, that the YB49 has indeed a high drag and that it varies little with the mach numbers (about 10% between 0.2 and 0.8 Mach).
BTW, the Nasa totally confirms to the free choise of reference area:
"Notice that the area (A) given in the drag equation is given as a reference area. The drag depends directly on the size of the body. Since we are dealing with aerodynamic forces, the dependence can be characterized by some area. But which area do we choose? If we think of drag as being caused by friction between the air and the body, a logical choice would be the total surface area of the body. If we think of drag as being a resistance to the flow, a more logical choice would be the frontal area of the body that is perpendicular to the flow direction. And finally, if we want to compare with the lift coefficient, we should use the same wing area used to derive the lift coefficient. Since the drag coefficient is usually determined experimentally by measuring drag and the area and then performing the division to produce the coefficient, we are free to use any area that can be easily measured. If we choose the wing area, rather than the cross-sectional area, the computed coefficient will have a different value. But the drag is the same, and the coefficients are related by the ratio of the areas. In practice, drag coefficients are reported based on a wide variety of object areas. In the report, the aerodynamicist must specify the area used; when using the data, the reader may have to convert the drag coefficient using the ratio of the areas." (https://www.grc.nasa.gov/www/k-12/airplane/drageq.html)
So, you still havent answered, why the YB49 has twice the drag of the B47 despite having the same wetted area. Its a simple qustion, why?
If you dont trust these proffessors, you could as well read that link: http://www.dept.aoe.vt.edu/~mason/Mason_f/NorthropYB-49.pdf which confirms, that the YB49 has indeed a high drag and that it varies little with the mach numbers (about 10% between 0.2 and 0.8 Mach).
BTW, the Nasa totally confirms to the free choise of reference area:
"Notice that the area (A) given in the drag equation is given as a reference area. The drag depends directly on the size of the body. Since we are dealing with aerodynamic forces, the dependence can be characterized by some area. But which area do we choose? If we think of drag as being caused by friction between the air and the body, a logical choice would be the total surface area of the body. If we think of drag as being a resistance to the flow, a more logical choice would be the frontal area of the body that is perpendicular to the flow direction. And finally, if we want to compare with the lift coefficient, we should use the same wing area used to derive the lift coefficient. Since the drag coefficient is usually determined experimentally by measuring drag and the area and then performing the division to produce the coefficient, we are free to use any area that can be easily measured. If we choose the wing area, rather than the cross-sectional area, the computed coefficient will have a different value. But the drag is the same, and the coefficients are related by the ratio of the areas. In practice, drag coefficients are reported based on a wide variety of object areas. In the report, the aerodynamicist must specify the area used; when using the data, the reader may have to convert the drag coefficient using the ratio of the areas." (https://www.grc.nasa.gov/www/k-12/airplane/drageq.html)
So, you still havent answered, why the YB49 has twice the drag of the B47 despite having the same wetted area. Its a simple qustion, why?
Last edited:
I don’t start guessing around, what you want to say?The airfoils used (http://airfoiltools.com/airfoil/details?airfoil=naca653618-il) were indeed very thick, which comes to little surprise. For sufficient internal space and height, flying wings tend to use thick airfoils. As we see in the L/D chart, the wings lost efficiency when going from Mach 0.2 to Mach 0.8 but not dramatic, so with the difference in Mach numbers between the YB35 and YB49 being much smaller than 4, they would have worked nearly equal good for both airplanes. The high drag of the YB49 is not caused by a suddenly occurring flow separation at a certain Mach number, it is inherent in the design with the ultra-thick wing profile (= high frontal area).
I don't think you understand drag divergence, or much of anything else.
View attachment 674374
Again a simple question;
Wing A = 1ft chord 20% thick
Wing B = 2ft chord 8% thick
Which has more drag? Assume its a 65 series section.
Hi Nicknick,
That obviously was a demand that you should specify the version.
It goes without saying that I also need you to specify the source.
Whatever your data might be, it won't change anything about the correctness of the weights I stated, for the specific versions I stated, supported by sources I provided without even being reminded (twice).
Regards,
Henning (HoHun)
the maximum starting weight of the B47 is 100 tons, that more than that of the YB49. Despite the the starting weight of the B47 is higher, the key póint is, that the YB49 has more than twice the drag of the B47 with equal wetted area.
Note that I gave you an exact version of the B-47, which you failed to do.
That obviously was a demand that you should specify the version.
It goes without saying that I also need you to specify the source.
Whatever your data might be, it won't change anything about the correctness of the weights I stated, for the specific versions I stated, supported by sources I provided without even being reminded (twice).
Regards,
Henning (HoHun)
We could start a new thread about the weight, but here we are discussing about resitance in relation to frontal area/wetted area and some tons more or less really doesn t matter.
As Sianar posted, the wetted area of the B49 is 11300 sq ft. As I wrote before:
"As we see in this example, the B47 had a wetted area of 11300 square feet. I haven’t found anything about the wetted area of the Yb49, but the wing area was 4000 square feet. The wetted area must have been more than twice as much, I guess something between 10.000 sq ft und 12.000 sq feet, which is quite similar to that of the B47"
So, it is totally plausibel, that both planes have about the same wetted area an my link is totally sound.
So why does the YB49 have about twice the drag ot the B47? As seen in my other link, the Mach number isnt an explanation.
As Sianar posted, the wetted area of the B49 is 11300 sq ft. As I wrote before:
"As we see in this example, the B47 had a wetted area of 11300 square feet. I haven’t found anything about the wetted area of the Yb49, but the wing area was 4000 square feet. The wetted area must have been more than twice as much, I guess something between 10.000 sq ft und 12.000 sq feet, which is quite similar to that of the B47"
So, it is totally plausibel, that both planes have about the same wetted area an my link is totally sound.
So why does the YB49 have about twice the drag ot the B47? As seen in my other link, the Mach number isnt an explanation.
Hi Nicknick
You're accusing me of posting false weight numbers, and now you're trying to dodge the request to specify the source for your claims? :-D
That's what one calls "double standards".
Sorry, I have to know now ... where are your 100 tons from, and for which version are they valid? Did you think I was making up numbers because that's what you are doing? :-D
Regards,
Henning (HoHun)
We could start a new thread about the weight, but here we are discussing about resitance in relation to frontal area/wetted area and some tons more or less really doesn t matter.
You're accusing me of posting false weight numbers, and now you're trying to dodge the request to specify the source for your claims? :-D
That's what one calls "double standards".
Sorry, I have to know now ... where are your 100 tons from, and for which version are they valid? Did you think I was making up numbers because that's what you are doing? :-D
Regards,
Henning (HoHun)
I just used Google an I found several sources which confirmed a take off weight of about 100 ton, its easy, just do it!
As said, the YB49 would have been much more efficient at greater height or slower speed, since it wasn’t flying with the optimum angle of attack (to low).
But even if we concider a lower take off weight, would it change anything written here about the parasitic drag vs. wetted area? Absolutly nothing!
As said, the YB49 would have been much more efficient at greater height or slower speed, since it wasn’t flying with the optimum angle of attack (to low).
But even if we concider a lower take off weight, would it change anything written here about the parasitic drag vs. wetted area? Absolutly nothing!
Last edited:
Hi Nicknick,
The principle of constructive discussion is, you make a claim, you prove it.
More bluntly: Put up or shut up.
I am quite willing to accept speculation or uncertain sources from people who are obviously making a honest effort to approach the facts as good as they can, and are transparent about what is what.
If you think that you can accuse me of making false claims here when everything I wrote was clearly sourced, and that you don't need to apologize when I point out to you your accusation was completely unwarrranted, but you yourself can claim whatever you want without any obligation to support that at all ... well, then I'm unable to count you into the "honest effort" category.
To be frank, I've had more constructive and mature discussions with hyperactive 4-year olds about how long they are allowed to stay up beyond bedtime.
Regards,
Henning (HoHun)
I just used Google an I found several sources which confirmed a take off weight of about 100 ton, its easy, just do it!
The principle of constructive discussion is, you make a claim, you prove it.
More bluntly: Put up or shut up.
I am quite willing to accept speculation or uncertain sources from people who are obviously making a honest effort to approach the facts as good as they can, and are transparent about what is what.
If you think that you can accuse me of making false claims here when everything I wrote was clearly sourced, and that you don't need to apologize when I point out to you your accusation was completely unwarrranted, but you yourself can claim whatever you want without any obligation to support that at all ... well, then I'm unable to count you into the "honest effort" category.
To be frank, I've had more constructive and mature discussions with hyperactive 4-year olds about how long they are allowed to stay up beyond bedtime.
Regards,
Henning (HoHun)
So you are running out of arguments and make a retreat. Youre final straw was beginning a discussion about take off weight, which is of absolutely no relevance when discussing parasitic drag. You could have ended this discussion much earlier, but you didn’t. You continued until you totally rund out of arguments.
- Joined
- 27 December 2005
- Messages
- 17,349
- Reaction score
- 23,979
Topic is locked as it seems to be in a death spiral. This is the end of the discussion.
Last edited:
- Status
- Not open for further replies.
Similar threads
-
-
-
WCarl Roe Variable-Geometry (Folding-/ Retractable-Wing) Patent 1942 (US2410239).
- Started by Wingknut
- Replies: 2
-
How release altitude affect the range of subsonic cruise missile with high glide ratio?
- Started by Ronny
- Replies: 5
-
A WW2 fighter design for rugged areas
- Started by Pasoleati
- Replies: 268

