Tundra Satellite System Capabilities

[panzerfeist1]

Tundra satellites for the EKS constellation

Tundra satellites for the Russian early warning system, EKS, by Anatoly Zak

www.russianspaceweb.com

"TsNII Kometa seemingly confirmed the modular design of the EKS satellite inherited from RKK Energia's Viktoria and Yamal projects. The company also said that the satellite would be capable of transmitting high volumes of information via special communications channels protected from interference. The satellite itself would also take over a great deal of data processing, which previously had to be conducted on the ground. Finally, the spacecraft would also carry an additional "informational" or communications function, apparently with the primary purpose of sending firing orders to the Russian strategic missile forces."

"According to RKK Energia, its satellites could detect targets climbing as high as 1,000 kilometers above the Earth surface (i.e. considerably higher than most ballistic missiles would normally fly). Detectable targets included hypersonic vehicles, strategic bombers, maneuvering low-orbital satellites and space junk orbiting as high as 36,000 kilometers above the Earth surface. A fire on Earth could be detected in 25 seconds, the company stressed. The satellite would sport sensors operating in five spectral ranges: ultraviolet, optical, and three types of infra-red light. They would reach a resolution of one meter."

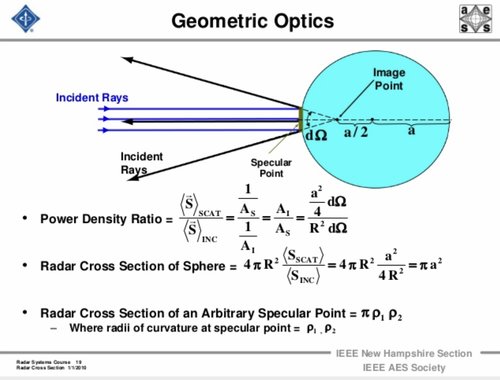
Satellite Radars would be out of the question.





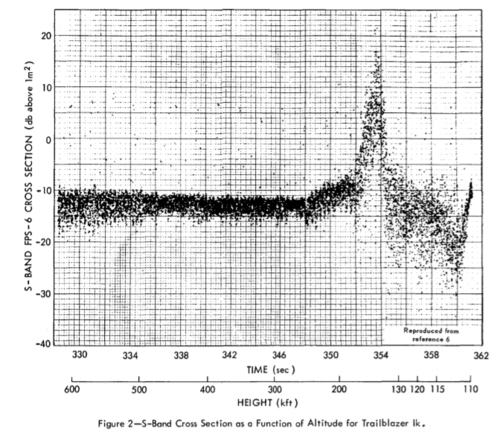
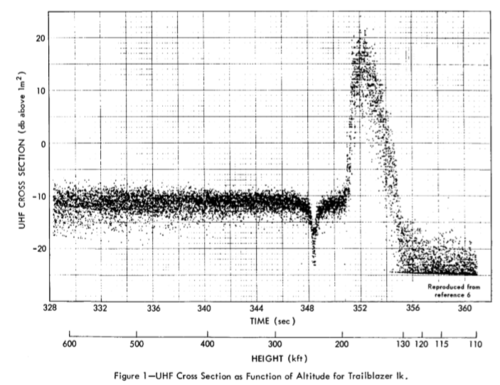
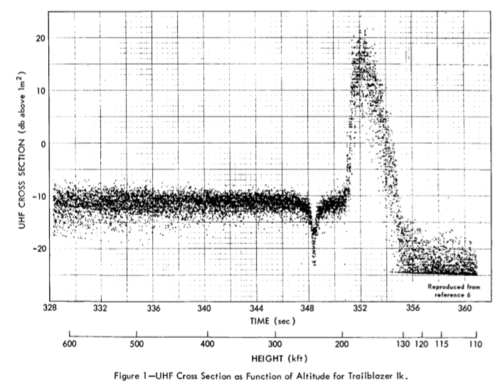
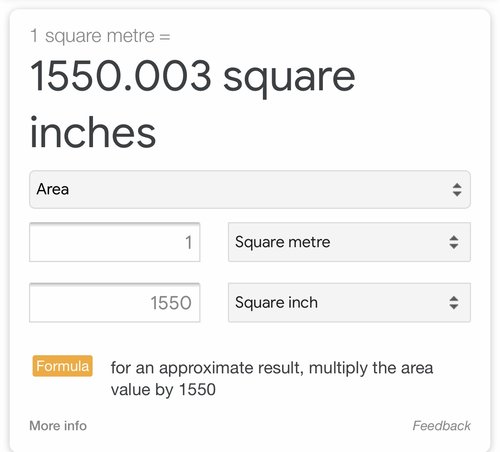
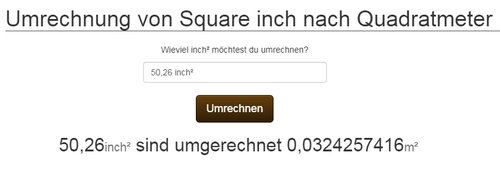
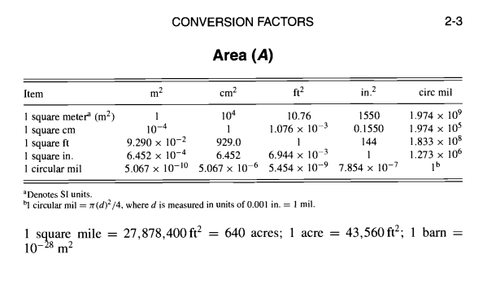
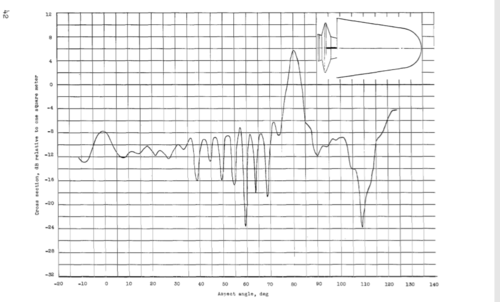
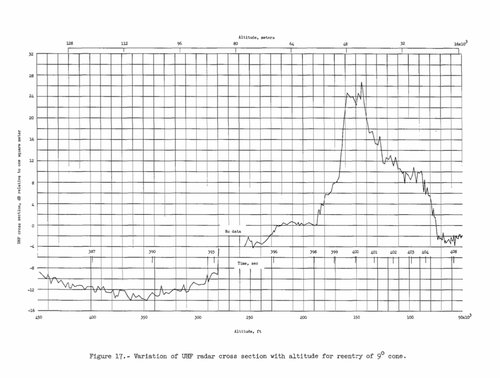
130,000ft to 110,000ft or 39.624kms to 33.528kms seems to effect the radar cross section in different radar spectrums of a hypersonic target so it would be problematic having missiles just staying in those altitudes. Is there any reason why scram or ramjets are not included for their space satellites as targets? I know that there are other future LEOs that include HGVs or use an image as a reference to their source when suggesting hypersonic weapons? Are they just low enough and have the right fast speed to make detection or tracking difficult? Because there are aerial targets they included like bombers which fly below supersonic speeds at lower altitudes but why not targets at slightly higher altitudes with a very good infrared signature? They also include HGVs but they are at higher altitudes than air-breathable missiles.

 

[Ronny]

From that link, it doesn't sound like Tundra Satellite uses radar as a part of its sensors, and they include hypersonic vehicles in their list of detectable targets. Ramjet, scramjet missiles and hypersonic glider should all count as hypersonic vehicles.

The satellite would sport sensors operating in five spectral ranges: ultraviolet, optical, and three types of infra-red light

Detectable targets included hypersonic vehicles, strategic bombers, maneuvering low-orbital satellites and space junk orbiting as high as 36,000 kilometers above the Earth surface

Tundra satellites for the EKS constellation

Tundra satellites for the Russian early warning system, EKS, by Anatoly Zak

www.russianspaceweb.com

But let say Tundra Satellite has a radar, the RCS reduction by plasma sheath on hypersonic missile won't put its radar out of the question.
The RCS chart belong to Trailblazer Ik which is an 8 inches diameter aluminum sphere with re entry speed of 19000 feet/second (Mach 16.8)
8 inches = 0.2 meter diameter, the radius is then 0.1 meter. A metal sphere with radius of 0.1 meters will have RCS of 0.03 m2 (-15 dBsm)
Trailblazer Ik already has low RCS without any plasma effect.

Radar RCS calculator | Radar RCS formula or equation

This page covers Radar RCS calculator.It mentions Radar RCS formula or equation used in this calculator.RCS stands for Radar Cross Section.

www.rfwireless-world.com

This is confirmed and described again by their words and the chart

above the earth's atmosphere, the free space cross section of the reentering object is seen. As the object descends, a high altitude decrease in cross section is observed at height of roughly 250,000 feet

This tells us the UHF radar cross section value of -18 to -10 dBsm ( 0.01-0.1 m2) we see at the altitude from 600 kft to 250 kft is the radar cross section of Trailblazer Ik without any reduction due to the plasma sheath. The plasma sheath bring the RCS down to -25 dBsm (0.003 m2) at 250 kft altitude. So it can reduce RCS by about 10 times.
Then next paragraph:

Following this dip, a period of relatively constant radar return may occur due, presumably, to the formation of a strongly-reflecting ionized sheath about the object. Next, the presence of a wake of sufficiently strong ionization results in a marked enhancement of the reflected radar signal. As reflections from the wake disappear at, say, 150,000 feet, a second decrease of radar cross section below the free space value is sometimes observed

As the sphere descended, the free space cross section was measured until an altitude of 240,000' feet was reached at which point an order of magnitude decrease in cross section took place. This was followed, after a period, by a large enhancement due to the wake.

So the huge spike enhancement that bring the radar cross section to 15 dBsm (31 m2) between 235 kft and 150 kft altitude is due to the strongly reflecting plasma sheath at sufficient ionization. Below 150 kft, a second decrease of radar cross section is sometime observed. Between 130kft -110 kft, the plasma sheath can reduce Trailblazer Ik RCS from the free space value of -16 dBsm down to -30 dBsm at UHF. It can reduce Trailblazer Ik RCS from the free space value of -16 dBsm down to -25 dBsm in S-band.

Radar RCS calculator | Radar RCS formula or equation

This page covers Radar RCS calculator.It mentions Radar RCS formula or equation used in this calculator.RCS stands for Radar Cross Section.

www.rfwireless-world.com

The UHF radar used in the experiment operates at 420 MHz, the S-band radar used in the experiment operates at 2.8 GHz
Trailblazer Ik reentry speed is 19,000 feet per second (Mach 16.88)
Trailblazer 1b reentry speed is 14,000 feet per second (Mach 12.44)
Trailblazer is an 8 inches diameter aluminum sphere
Hypersonic missiles are a lot bigger than Trailblazer so their rcs should be bigger unless they use shaping and material to reduce it. But traditional material and stealth shape doesn't work well with hypersonic speed.
Scramjet and ramjet missiles are slower than Trailblazer so their plasma frequency isn't as high that should reduce their effectiveness compared to Trailblazer against higher frequency
And satellite can use higher frequency radar such as X-band, J-band, I-band, all are highly immune to the plasma sheath created by hypersonic missile

 

[GARGEAN]

...

I wouldn't put too much faith into that sphere RCS calculator. What it does is just calculates circle area, lol. While RCS is quite a bit more complicated function than visual area of irradiated object.

 

[Ronny]

I wouldn't put too much faith into that sphere RCS calculator. What it does is just calculates circle area, lol. While RCS is quite a bit more complicated function than visual area of irradiated object.

Sure, it cant calculate the exact value, only the approximation of max RCS of a perfectly conducting sphere. I use that as an illustration tool

For the true free space rcs of trailblazer, you can see in the NASA chart:
Like they said: 600 kft-240kft altitude is the free space RCS of Trailblazer Ik without plasma cloak
Below 240 kft-230kft altitude is the RCS of Trailblazer Ik with plasma sheath acts as absorbing layer
Below 230 kft-150 kft altitude is the RCS of Trailblazer after it is enhanced by the plasma sheath
Below 130kft -110 kft, plasma sheath has some absorbing ability again.

 

[GARGEAN]

only the approximation of max RCS of a perfectly conducting sphere.

Yeah. Which ends up to be just area of projection of said sphere but as you said quite useless for IRL due to perfect sphere of perfect material in perfectly lossless space with sphere size/wavelengh approaching infinity... Why they even used that "RCS calculator" logo?

 

[panzerfeist1]

But let say Tundra Satellite has a radar, the RCS reduction by plasma sheath on hypersonic missile won't put its radar out of the question.
The RCS chart belong to Trailblazer Ik which is an 8 inches diameter aluminum sphere with re entry speed of 19000 feet/second (Mach 16.8)
8 inches = 0.2 meter diameter, the radius is then 0.1 meter. A metal sphere with radius of 0.1 meters will have RCS of 0.03 m2 (-15 dBsm)
Trailblazer Ik already has low RCS without any plasma effect.

There are 3 problems here and I believe Gargean addressed one of them.
1st Problem is your mathematical input.
Since formulas do not give a sh$% if you are using meters or inches I will put 4 inches instead, ignore the fact the calculator says meters that will be converted later. I get 50.26548245743669inchm2, than I convert 1 meter to inches and get 39.3701inches. Now to get my estimates back to meters I divide that 50 number by that 39 number I get 1.276742564977907m2...… either way dropping from a constant -10 to -25 decibels is not that bad in S-band or -10 to -23 decibels in UHF and the 2nd decrease all the way to -25 decibels.

2nd problem is material of the object. Such as does gold have a higher reflective property than a aluminum?

3rd problem which I believe Gargean addressed is wavelengths. Example there is a source of a Chinese missile
that according to the Nebo-M sees the missile as .002m2 in X-band but with UHF it sees the missile as a 0.6m2 target.

Trailblazer is an 8 inches diameter aluminum sphere
Hypersonic missiles are a lot bigger than Trailblazer so their rcs should be bigger unless they use shaping and material to reduce it.

Shape and materials matters F-22 may appear to have a lot of surface area in the front, but radars see its surface area as a steel marble

And satellite can use higher frequency radar such as X-band, J-band, I-band, all are highly immune to the plasma sheath created by hypersonic missile

If there frequencies are high enough to remain unaffected by the plasma frequency. Example is your kempster source.

 

[Ronny]

There are 3 problems here and I believe Gargean addressed one of them.
1st Problem is your mathematical input.
Since formulas do not give a sh$% if you are using meters or inches I will put 4 inches instead, ignore the fact the calculator says meters that will be converted later. I get 50.26548245743669inchm2, than I convert 1 meter to inches and get 39.3701inches. Now to get my estimates back to meters I divide that 50 number by that 39 number I get 1.276742564977907m2...… either way dropping from a constant -10 to -25 decibels is not that bad in S-band or -10 to -23 decibels in UHF and the 2nd decrease all the way to -25 decibels.

2nd problem is material of the object. Such as does gold have a higher reflective property than a aluminum?

3rd problem which I believe Gargean addressed is wavelengths. Example there is a source of a Chinese missile
that according to the Nebo-M sees the missile as .002m2 in X-band but with UHF it sees the missile as a 0.6m2 target.

1st: You convert incorrectly.
1 meter = 39.37 inches
But 1 square meter = 1550 square inches.
This is a good and simple explanation of the problem:

So 50.26 square inches = 0.0324 square meters

2nd: The equation assumes a perfectly conducting sphere, so a real sphere with less than perfect conducting property will have a lower radar cross section

3rd: That correct, due to the size of the object compared to the wave, you will have reflection property in Optical, Mie or Ray length region. But if you don't trust the estimate value, you can take number from NASA's chart: 600 kft-240kft altitude is the free space RCS of Trailblazer Ik without plasma cloak. So Trailblazer Ik has radar cross section value of -18 to -10 dBsm ( 0.01-0.1 m2) in free space.

Shape and materials matters F-22 may appear to have a lot of surface area in the front, but radars see its surface area as a steel marble

That is correct, but hypersonic vehicle doesn't have the luxury of aircraft, they have to deal with heating, drag, expansion at several orders of magnitude greater.

If there frequencies are high enough to remain unaffected by the plasma frequency. Example is your kempster source.

Yes, that is the point. Logically, satellite and airborne radar uses high frequency radar because they can use smaller antenna with high frequency.

 

[Ronny]

Yeah. Which ends up to be just area of projection of said sphere but as you said quite useless for IRL due to perfect sphere of perfect material in perfectly lossless space with sphere size/wavelengh approaching infinity... Why they even used that "RCS calculator" logo?

To estimate the rough max value, real value is lower due to the less than perfect conducting property.

 

[panzerfeist1]

1st: You convert incorrectly.
1 meter = 39.37 inches
But 1 square meter = 1550 square inches.
This is a good and simple explanation of the problem: So 50.26 square inches = 0.0324 square meters

You are still trying to stiff me. After using 4 inches for radius it turns out to be 50.26548245743669inchm2 and since I am trying to convert this to "meters squared" I will have to divide it by 39.3701inchesm2(instead of just inches) and the results will be just the same in meters squared.

Your trying to square a decibel number which will obviously result in a very small number for that formula, but squaring a number that is 1 or above that will result in a bigger number than the number that was put on the radius.

A very simple example of what I mean which you are exactly doing the same way but different style. 2 feet times 2 feet we get 4 feet, We see the length of another square being 8 inches and width being 8 inches, We are trying to solve how much 8 by 8 inches squares can fit inside 2 ft by 2 ft square.

There is a guy named Ronny that says multiply the inches, convert it to feet, than divide it by the 4ft square his answer is .75 and he concludes that the 8 inch by 8 inch box is too big for the 2 ft by 2f box

There is a guy named panzerfeist that decides to turn the 4 ft square measurement into 24 by 24 inches instead and his results are 576 inches he than divides that by 64 inches from the 8 inch by 8 inch square his results are nine 8 inch by 8 inch squares can fit inside the 2 by 2ft square.

I used the same exact method with the sphere, I only used the inches formula for my square and sphere example, instead of meters(or feet with both my ronny examples) I stayed with inches and my output was inches squared for the sphere and for my square, while somehow Ronny is trying to figure out why the area for his square or sphere is too small of a calculation for his output when the values of his inputs are much larger than his own area calculations. This is not a mockery considered it a lesson when using metrics.

2nd: The equation assumes a perfectly conducting sphere, so a real sphere with less than perfect conducting property will have a lower radar cross section

Where does it say in the source assume a good conducting sphere? A simple google search is telling me that alluminum attenuates certain radio signals. I am just saying materials are important

 

[Ronny]

You are still trying to stiff me. After using 4 inches for radius it turns out to be 50.26548245743669inchm2 and since I am trying to convert this to "meters squared" I will have to divide it by 39.3701inchesm2(instead of just inches) and the results will be just the same in meters squared.

Your trying to square a decibel number which will obviously result in a very small number for that formula, but squaring a number that is 1 or above that will result in a bigger number than the number that was put on the radius.

I am not trying to stiff you, whatever that supposed to mean. This is elementary maths.
In your last post:
you put the radius of the square as 4 inches, so you get area equal 50.26 square inches.
Then you convert 1 meter to inches and get 39.3701 inches
Then to get your estimates back to square meters you divide that 50 number by that 39 number you get 1.276742564977907m2
So you concluded that 50.26 square inches equal 1.2 square meters.
But I am telling you that you are doing it the incorrect way, so your answer is Wrong.
1 meter = 39.37 inches
But 1 square meter = 1550 square inches.
So to convert 50.26 square inches to square meters, you have to divide 50.26 by 1550. So the correct conversion is: 50.26 square inches equal 0.032 square meters.

A very simple example of what I mean which you are exactly doing the same way but different style. 2 feet times 2 feet we get 4 feet, We see the length of another square being 8 inches and width being 8 inches, We are trying to solve how much 8 by 8 inches squares can fit inside 2 ft by 2 ft square.

There is a guy named Ronny that says multiply the inches, convert it to feet, than divide it by the 4ft square his answer is .75 and he concludes that the 8 inch by 8 inch box is too big for the 2 ft by 2f box

There is a guy named panzerfeist that decides to turn the 4 ft square measurement into 24 by 24 inches instead and his results are 576 inches he than divides that by 64 inches from the 8 inch by 8 inch square his results are nine 8 inch by 8 inch squares can fit inside the 2 by 2ft square.

I used the same exact method with the sphere, I only used the inches formula for my square and sphere example, instead of meters(or feet with both my ronny examples) I stayed with inches and my output was inches squared for the sphere and for my square, while somehow Ronny is trying to figure out why the area for his square or sphere is too small of a calculation for his output when the values of his inputs are much larger than his own area calculations. This is not a mockery considered it a lesson when using metrics.

This is the exact opposite of what is going on.

Where does it say in the source assume a good conducting sphere? A simple google search is telling me that aluminum attenuates certain radio signals. I am just saying materials are important

Because it uses the base equation to calculate sphere RCS. Same for the base equation used to estimate RCS of flat plate, cylinder. The object are always assumed to be perfect conducting. Otherwise, it can't be used as the base equation anymore. The aluminum of the real Trailblazer might attenuate certain radio signals but that only makes the total RCS lower than the estimated number. If the real number is smaller then it only proves my point more.

 

[panzerfeist1]

So you concluded that 50.26 square inches equal 1.2 square meters.
But I am telling you that you are doing it the incorrect way, so your answer is Wrong.
1 meter = 39.37 inches
But 1 square meter = 1550 square inches.
So to convert 50.26 square inches to square meters, you have to divide 50.26 by 1550. So the correct conversion is: 50.26 square inches equal 0.032 square meters.

2nd attempt to stiff me.
one square meter is equal to 39.37 inches x 39.37 inches in other words 1m2 is 39.37 inches squared. 1549.9969 square inches or rounded off 1550 square inches......In other words you forgot to square the 50 number(or multiply 50 by 50) to give you the numerical result than dividing that by 39 inches squared or in simpler terms 39 times 39.

If the real number is smaller then it only proves my point more.

I am a little suspicious about the S-band and UHF cross section with the parenthesis of (decibels above 1m2) However I cannot determine where they have dropped the spheres, was 660,000ft recorded because that was their plans to start the radar recordings, or their radars cannot detect the objects above 200kms, etc. But I am assuming it is -10 decibels because that's what it starts off as.







I know you have an account here and an account at the other forum. But just 2 questions, how come you are liking stuff key forum pub related when I have never seen anyone named Ronny in that place. Also how come you do not have an account at f-16.net? I have one user over there that mentions this forum, that same user told me he is mig-31bm at key forum pub, I asked if those were your accounts and you actively denied it. So how come you have not started an account at F-16.net and what username did you have at key forum pub?

 

[Ronny]

2nd attempt to stiff me.
one square meter is equal to 39.37 inches x 39.37 inches in other words 1m2 is 39.37 inches squared. 1549.9969 square inches or rounded off 1550 square inches......In other words you forgot to square the 50 number(or multiply 50 by 50) to give you the numerical result than dividing that by 39 inches squared or in simpler terms 39 times 39.

Omfg, no one is trying to stiff you.
If I am lying to you then why Google converter has the same result as I told you?. Do you think I hack into Google server to deceive you?
A square with each edge length equal 39.37 inches will have an area equal 1576 square inches instead of 39.37 square inches. Or in simpler term, 39 times 39 doesn't equal 39.

I am a little suspicious about the S-band and UHF cross section with the parenthesis of (decibels above 1m2) However I cannot determine where they have dropped the spheres, was 660,000ft recorded because that was their plans to start the radar recordings, or their radars cannot detect the objects above 200kms, etc. But I am assuming it is -10 decibels because that's what it starts off as

It is explained very clear in the paragraph I post earlier.

View attachment 623544



View attachment 623545

I know you have an account here and an account at the other forum. But just 2 questions, how come you are liking stuff key forum pub related when I have never seen anyone named Ronny in that place. Also how come you do not have an account at f-16.net? I have one user over there that mentions this forum, that same user told me he is mig-31bm at key forum pub, I asked if those were your accounts and you actively denied it. So how come you have not started an account at F-16.net and what username did you have at key forum pub?

Do you always play victim because you have short term memory lost?. I told you this is the third time that I have eloise account in F-16.net and mig-31bm in keypubforum. It is in the topic less than a week ago: Read #4.

https://www.secretprojects.co.uk/threads/plasma-stealth-on-missiles.32768/

 

[panzerfeist1]

Omfg, no one is trying to stiff you.
If I am lying to you then why Google converter has the same result as I told you?. Do you think I hack into Google server to deceive you?
A square with each edge length equal 39.37 inches will have an area equal 1576 square inches instead of 39.37 square inches. Or in simpler term, 39 times 39 doesn't equal 39.

How many inches is equivalent to 1 square meter?

Joe Shuffield's answer: one square meter is 39.37 inches x 39.37 inches (there are 39.37 inches in a meter) so the answer is 1549.9969 square inches or rounded off 1550 square inches

qr.ae

How many inches is equivalent to 1 square meter?

W. M. H. Dayaratna's answer: Your question is wrong . Inches measure length and square meter measures area. You can’t equal these two dimensionally. It should be How many square inches is equivalent to one square meter? Answer: 1m = 39.3 inch 1m2 = (39.3)2 inch2 = 1544.49 inch2

qr.ae

So am I correct for multiplying the 50 inches by 50 inches output for 50inches squared, and for converting 1 square meter to 39 inches squared, than dividing 50 inches squared by 39 inches squared?

Do you always play victim because you have short term memory lost?. I told you this is the third time that I have eloise account in F-16.net and mig-31bm in keypubforum. It is in the topic less than a week ago: Read #4.
https://www.secretprojects.co.uk/threads/plasma-stealth-on-missiles.32768/

I must have ignored it,

 

[Ronny]

Omfg, no one is trying to stiff you.
If I am lying to you then why Google converter has the same result as I told you?. Do you think I hack into Google server to deceive you?
A square with each edge length equal 39.37 inches will have an area equal 1576 square inches instead of 39.37 square inches. Or in simpler term, 39 times 39 doesn't equal 39.

How many inches is equivalent to 1 square meter?

Joe Shuffield's answer: one square meter is 39.37 inches x 39.37 inches (there are 39.37 inches in a meter) so the answer is 1549.9969 square inches or rounded off 1550 square inches

qr.ae

How many inches is equivalent to 1 square meter?

W. M. H. Dayaratna's answer: Your question is wrong . Inches measure length and square meter measures area. You can’t equal these two dimensionally. It should be How many square inches is equivalent to one square meter? Answer: 1m = 39.3 inch 1m2 = (39.3)2 inch2 = 1544.49 inch2

qr.ae

So am I correct for multiplying the 50 inches by 50 inches output for 50inches squared, and for converting 1 square meter to 39 inches squared, than dividing 50 inches squared by 39 inches squared?

Don't you see that they are telling the samething I am telling you?
1 square meter is 1544 square inches. So it is obvious that your 50.4 square inches doesn't equal to 1.2 square meter.

 

[panzerfeist1]

So it is obvious that your 50.4 square inches doesn't equal to 1.2 square meter.

50.26548245743669inches2 are you pretending that you have forget that all the answers in that calculator came out squared? I converted 1 meter squared to 39 inches squared for the same numerical value. and divided the 50 inches squared by 39 inches squared. How is this difficult to understand?

 

[Ronny]

50.26548245743669inches2 are you pretending that you have forget that all the answers in that calculator came out squared?

Square represent the area.
50.26 inches2 does not equal 1.2 m2
50.26 inches2 equal 0.032 m2
Is that clear enough for you?.
You can ask quora or anywhere on this planet Earth, you will get the same answer as I wrote above.

 

[panzerfeist1]

50.26 inches2 does not equal 1.2 m2

no shit I divided the the 50 inches squared by 39 inches squared because I know that 39 inches squared = 1m2

50.26 inches2 equal 0.032 m2

dont forget to square the 50 and square the 39.

 

[Nigelhg]

I can't believe what I'm reading- seriously.... Panzer please take a basic math course before you make yourself look even more idiotic.

Step back have a think.

39inches*39inches = 1521 inch2

Length inches X length inches = area inch2

39 square inches = 39 inch2.

This is how area is expressed in maths.

You don't square again

 

[Ronny]

because I know that 39 inches squared = 1m2

I dare you find a converter anywhere on this planet earth that give you "39 square inches = 1 square meter"

 

[panzerfeist1]

I can't believe what I'm reading- seriously.... Panzer please take a basic math course before you make yourself look even more idiotic.

Step back have a think.

39inches*39inches = 1521 inch2

Length inches X length inches = area inch2

39 square inches = 39 inch2.

This is how area is expressed in maths.

You don't square again

Atleast bother using Ronny's calculator please. if 1 meter come outs squared as multiplying 39 by 39 inches, than inches come out squared by multiply 50 by 50, if inches come out as inches than the answer would be as inches and if meters come out as meters than the answer will be in meters.....Mind blown

I guess they do not call f-16.net a cult for no reason. Ironically the biggest idiots I view there only have accounts over there but no multiple accounts on other forums for a very good reason, its like a containment board for those kind of people as /pol/ is for 4chan.

I dare you find a converter anywhere on this planet earth that give you "39 square inches = 1 square meter"

1 square meter is 39 times 39 if you want to double the end results for inches to get square meters, than you do the same for 50 inches.

Also the 2nd reason why I am right is that you are intentionally multiply the values as decimals and any user here that is not mentally challenged with an account here will tell you it does not make sense for the area of a square, sphere, cylinder to be smaller than the radius, length, width, etc...….In fact I believe no one in grade school ever multiplied decimals for the areas above here. I don't even remember my teacher doing decimal multiplication for the areas above. Its like putting 0.25 ft for 3 inches(like you put 0.2 meters for 4 inches) and to solve the length and width of .25 ft box you get .0625 ft convert that to inches you get an area of 3/4th of an inch. But my way of doing things is multiplying the 3 inch by 3 inch of the length and width of the box I get a 9 inch area. Who here is correct? Hint: The one that did not use decimals values.

3rd reason put 4 in radius we get 50 inches squared divided by 39 inches squared( because 1 meter squared equals this) you get 1.28 meters squared. 1.28 meters squared equals 2539.52499969 inches.

Do not forget that the output in that calculator is raised to the 2nd power. Put 4 meters you get 50 meters and 50 meters to the 2nd power you get like 2500 meters squared. put 4 inches you get 50 inches to the 2nd power, but you get upset when I put that 50 inch to the 2nd power. You don't want to put those inches to the 2nd power that is fine by me only if you don't put the meters to the 2nd power because that is a little unfair.

 

[bobbymike]

Trying to follow the thread but it appears the miscommunication is that 50 square inches being a hypothetical square 7.07x7.07 HxW correct? Now the meter by meter square equates to a 39.37 x 39.37 HxW square or 1550 square inches.

50/1550 = 0.0323

Or you can edge align each square and count horizontally where 7.07 fits 5.57 times from side to side and 5.57 times top to bottom. Squared you have a ~31.02 times bigger squared object OR the smaller square is ~0.0323 of the larger box

If I’m interpreting the original message correctly

 

[Ronny]

Also the 2nd reason why I am right is that you are intentionally multiply the values as decimals and any user here that is not mentally challenged with an account here will tell you it does not make sense for the area of a square, sphere, cylinder to be smaller than the radius, length, width, etc...….In fact I believe no one in grade school ever multiplied decimals for the areas above here. I don't even remember my teacher doing decimal multiplication for the areas above. Its like putting 0.25 ft for 3 inches(like you put 0.2 meters for 4 inches) and to solve the length and width of .25 ft box you get .0625 ft convert that to inches you get an area of 3/4th of an inch. But my way of doing things is multiplying the 3 inch by 3 inch of the length and width of the box I get a 9 inch area. Who here is correct? Hint: The one that did not use decimals values.

I don't know where did you study but there is no problem with multiplying by decimal.
If there is a square with edge length equal 10 cm.
You can find the area by multiply:
10*10 = 100 cm2
Or 0.1*0.1 = 0.01 m2 (this does not mean the area is smaller than the edge, length and area are 2 different concept)
Because 0.01 m2 = 100 cm2.
And for your information,
If you have a square with edge length of 0.25 feet, the area is 0.0625 square feet (also express as 0.0625 feet2). But area of 0.0625 square feet equal 9 square inches, it does not equal 0.75 square inches. Because, 1 square foot equal 144 square inches.

3rd reason put 4 in radius we get 50 inches squared divided by 39 inches squared( because 1 meter squared equals this) you get 1.28 meters squared.

Area of 39 square inches does not equal area of 1 square meter, and area of 50 square inches does not equal area of 1.28 square meter. Go check in any online converter.

 

[panzerfeist1]

I don't know where did you study but there is no problem with multiplying by decimal.

@bobbymike

There is a 1 meter box, and there is a separate box you want to fit in that 1 meter box that consists of 4 by 4 inches. Ronny says there is no problem using decimals so his calculations will be 0.2 meters times 0.2 meters to solve how many 4 by 4 inch boxes will fit in the 1 meter box. He gets .04 meters than divides that number by 1 meter. he concludes 24 4 by 4 inches fit in that 1 meter box.

Me on the other hand I multiply 4 by 4 inches and get 16 inches, than I multiply 39 by 39 inches and I get 1521 than I divide that number 1521 by 16 and I say 95 4 by 4 inches boxes can fit in that 1 meter box.

Who here is correct?

Area of 39 square inches does not equal area of 1 square meter, and area of 50 square inches does not equal area of 1.28 square meter. Go check in any online converter

you decided that 1m to the 2nd power is 39 times 39. I decided that 50 inches by the 2nd power in that output is around 2500. However I divide that 2500 by 1 meter to the 2nd power and get 1.28 meters squared.

 

[Ronny]

@bobbymike

There is a 1 meter box, and there is a separate box you want to fit in that 1 meter box that consists of 4 by 4 inches. Ronny says there is no problem using decimals so his calculations will be 0.2 meters times 0.2 meters to solve how many 4 by 4 inch boxes will fit in the 1 meter box. He gets .04 meters than divides that number by 1 meter. he concludes 24 4 by 4 inches fit in that 1 meter box.

How can you get such a simple multiplication wrong?
4 inches is only about 0.102 meter
Each 4*4 inches box will have area equal 16 square inches or about 0.0104 square meter. So about 96 of 4*4 inches box will fit in 1*1 meter box
That example of your is also the exact opposite of what is going on here.
You are the one who said: 39 square inches equal 1 square meter and that 50.26 square inches equal 1.2 square meters. Iam the one who said area of 50.26 square inches equal 0.032 square meters. And area of 39 square inches equal to 0.02516 square meter.
In simple words:
Your claim is that 1 m2 box can hold a single 39 inches2 box while I said 1 m2 box can hold about 39 of 39 inches2 box

 

[panzerfeist1]

So about 96 of 4*4 inches box will fit in 1*1 meter box

Now you are changing your story. You are the one that used a decimal for that calculator not me. I used numbers(not the metrics) than did the conversion for metrics at the end.

 

[Ronny]

Now you are changing your story. You are the one that used a decimal for that calculator not me. I used numbers(not the metrics) than did the conversion for metrics at the end.

I am still using decimal, it does not matter. 4 inches is about 0.102 meters. So area of each small box is 0.0104 square meters. So 1 square meter big box can contain: 1/0.0104= 96 small (4*4 inches) box

 

[panzerfeist1]

I am still using decimal, it does not matter.

Lets try it your way. I put .1 meters at radius and get .0314. I can also get that same value by doing 0.1 times 0.1 times 3.14........ I Put 4 times 4 times for the inches and times that with 3.14 I get 50.24(holy smokes the same results when I put 4 for radius for that calculator) than I do the conversion to meters. If you want to raise the meters to the 2nd power for meters square I will do the same with 50 than divide it.

 

[Ronny]

Lets try it your way. I put .1 meters at radius and get .0314. I can also get that same value by doing 0.1 times 0.1 times 3.14........ I Put 4 times 4 times for the inches and times that with 3.14 I get 50.24(holy smokes the same results when I put 4 for radius for that calculator)

No shit, that how it is calculated

than I do the conversion to meters. If you want to raise the meters to the 2nd power for meters square I will do the same with 50 than divide it.

You do the conversion incorrectly.
To convert 50.24 square inches to square meters, you have to divide 50.24 by about 1550 and you will get 0.0324 square meter. The "square" in "square inches" is how area is express in Maths. You don't get to raise the power a second time

 

[panzerfeist1]

No shit, that how it is calculated

Than why were you raising it the 2nd power the 2nd time for the output when it was already done the 1st time?

You do the conversion incorrectly.
To convert 50.24 square inches to square meters, you have to divide 50.24 by about 1550 and you will get 0.0324 square meter. The "square" in "square inches" is how area is express in Maths. You don't get to raise the power a second time

inches square vs. square inches

is "a few inches square" an equivalent phase to "a few square inches"? Example: this device is a few inches square

forum.wordreference.com

DIMENSION...............SURFACE AREA
1 inch square (1"x1") = 1 square inch
2 inches square (2"x2") = 4 square inches
3 inches square = 9 square inches
4 inches square = 16 square inches

The results do not come out meters square in that calculator, they come out square meters. In other words you have no reason to pull out 1550 without pulling 2500 as well.

So Ronny what happens when you divide 50 square inches with 1 square meter?



What is the point of using this useless calculator if you simply square the radius and multiply 3.14 while missing the other variables? Time for me to seal the deal with this image.

Therefore I am not in the wrong for dividing 50 inches by 39 inches. I believe that you have told me 1m2 is 1550 inches correct? Do you want to convert those 1550 inches to meters or square meters to verify that it turns out as 1m2? I believe if you and I had very similar interests in topics we would be holding hands and skipping because I believe our different interests through different forums just shows we cant get along. If you think I am wrong or that my calculations are still wrong for pulling this table than let me know. We need to go our separate ways sooner or later after this discussion here is done with.

 

[Ronny]

Than why were you raising it the 2nd power the 2nd time for the output when it was already done the 1st time?

What second power are you talking about?
Why did I divided 50.24 square inches by 1550 to get square meter? Because 1 square meter is 1550 square inches.

inches square vs. square inches

is "a few inches square" an equivalent phase to "a few square inches"? Example: this device is a few inches square

forum.wordreference.com

DIMENSION...............SURFACE AREA
1 inch square (1"x1") = 1 square inch
2 inches square (2"x2") = 4 square inches
3 inches square = 9 square inches
4 inches square = 16 square inches

The results do not come out meters square in that calculator, they come out square meters. In other words you have no reason to pull out 1550 without pulling 2500 as well.

Do you know what square meter mean in area?
When someone say: the area of a circle is 1550 square inches, then what he mean is the area is 1550 inches2.
The calculator give you answer in m2, if you replace meters by inches in the input then to convert the result to m2, you need to divide by 1550.

So Ronny what happens when you divide 50 square inches with 1 square meter?

You get 0.0324
Each 50 square inches box equal to 0.0324 time of 1 square meter box. In short, 1 square with area of 1 square meter can hold about 30 of these small square if the area of each small square is 50 square inches

View attachment 623554

What is the point of using this useless calculator if you simply square the radius and multiply 3.14 while missing the other variables?

Check the equation to calculate sphere RCS.

Time for me to seal the deal with this image.

View attachment 623556 Therefore I am not in the wrong for dividing 50 inches by 39 inches. I believe that you have told me 1m2 is 1550 inches correct? Do you want to convert those 1550 inches to meters or square meters to verify that it turns out as 1m2?

1 m2 is 1550 inches2, in words: 1 square meter equal to 1550 square inches. I have no idea how could you think that image prove your point when I have given you the Google converter result.

 

[panzerfeist1]

What second power are you talking about?
Why did I divided 50.24 square inches by 1550 to get square meter? Because 1 square meter is 1550 square inches.

Why is this graph saying that you are wrong? How come it says 1 meter =1m2 when we know one meter is 39 inches? Have you divided the 152o inches back to meters to confirm what you are saying is true? If you believe that the area is 1m2 = 1520 inches, than 1m2 also equals 39 meters. And no f*^(king 1m2 box would give an area of 39 meters..... That is impossible.

Do you know what square meter mean in area?
When someone say: the area of a circle is 1550 square inches, then what he mean is the area is 1550 inches2.
The calculator give you answer in m2, if you replace meters by inches in the input then to convert the result to m2, you need to divide by 1550.

39 times 39 times 3.14 we get 4778.362426110075 inches squared. So to get this back to meters squared for a sphere we divide this number back to 39 times 39 times 3.14 to get 1m2 back. Not to add any intentional bad humor to this but you are solving the area for a sphere and after you solve the area for the sphere you think its ok to also to go solve the area of a square next and believe that its is all but one equation. 4 inches times 4 inches times 3.14 will stay as 50 inch2 sphere 1 m times 1m times 3.14 will stay as 3.14m2 sphere. 123.622 inches is in the 3.14 meter sphere, 50 inches is in the other sphere. divide the 50 inch sphere by the 123 inch sphere... To put this in layman terms you are asking to fit a sphere insided a square for your conversion. But I am fitting a 50 inch area sphere to a 3.14 meter area sphere.

ach 50 square inches box equal to 0.0324 time of 1 square meter box.

We are not solving for boxes but spheres instead.

1 square meter equal to 1550 square inches

If thats the case convert the square inches back to meters and see if that lines up with one square meter. I am sure your answer will be no.

 

[Deino]

@panzerfeist1 ... sorry to step in but math seems to be quite simple and not depending any additional ideas

As such I don't know how you come to and still try to explain why 50.26 square inches are NOT by your calculation 0.032 square meters but 1.2 square meters ??

Just leave it ... by the way, it's Christmas time, and aren't there better things to do than trying to mend math?

 

[stealthflanker]

Well there is a conversion factor needs to be considered. Especially if you want to "translate" a unit into another. It is so weird that one need a whole long way when many simple tables and engineering books exist.

This is what i use to work with, conversion factor from book "AIAA Aerospace Design Engineers Handbook"

 

[zen]

A perfect sphere would only reflect a small amount of radar energy back towards the transmitter. Almost down to that part of the surface that is virtually flat to the source. Granted some energy might do a full loop back around the surface but only to then radiate outwards.

 

[panzerfeist1]

50.26 square inches are NOT by your calculation 0.032 square meters but 1.2 square meters ??

http://www.endmemo.com/cconvert/m2m.php Why does 1m2 = 1 meter and how come 1 meter =39 inches. With the inches calculation into consideration the area becomes 39 meters and 39 meters does not = 1m2.

 

[panzerfeist1]

@Ronny

I got newer better data that pertains more to the Zircon than it does to a sphere with frequencies included in the calculation regarding RCS.
https://ntrs.nasa.gov/archive/nasa/casi.ntrs.nasa.gov/19660006803.pdf (better article)

Vehicle
The Trailblazer I1 vehicle is essentially a four-stage solid-fuel rocket system with the motors arranged in tandem. The third and fourth stages are mounted in a structural shell (velocity package) and face in a rearward direc- tion at launch. view of the velocity package, in figure 3(b). During second-stage firing, the canted fins of the rocket spin the remaining system to maintain the attitude of the velocity package at approximately 650 (up from the launch horizontal) throughout the remainder of the flight. After second-stage firing, the velocity package separates and coasts to apogee. The complete vehicle is shown in figure 3(a), and a cut-away
When the velocity package has passed apogee, the third stage fires and pro- pels the third and fourth stages downward out of the open end of the structural shell. On the Trailblazer IIa (after third-stage burnout) a l5-inch (0.381 m) spherical rocket motor mounted in the rear of the payload was fired and accel- erated the fourth stage (payload) to approximately 19,400 ft/sec (5913.1 m/sec). The trajectory of the Trailblazer has been designed to bring the reentry of the payload back toward the launch site to facilitate radar measurements. Addi- tional information on the Trailblazer IIa vehicle can be obtained from refer- ence 11 which covers the performance of several Trailblazer I1 vehicles during flight.

Before the flight, the radar are calibrated to determine the relationship between the logarithmic intermediate-frequency-amplifier voltage pulses and the apparent radar-target cross section. Signals of known power are injected into the radar receiver and the corresponding logarithmic voltages of the intermediate-frequency amplifier are recorded and plotted to establish an S-shaped signal power-voltage curve for each radar. from a standard target at given slant ranges is determined for each radar by tracking a 6-inch-diameter (0.0015 m) aluminum sphere carried aloft by a bal- loon. This information provides all the variables necessary to calculate the value of the radar constant K from the simplified standard radar equation The signal power reflected
where u is the target cross-sectional area in square meters, P, is the received power in watts, and Rr is the slant range of the target from the radar in feet (meters). in reference 13. Further information on such calibrations can be found
The radar equation must be applied to each of the 320 readings per second to develop continuous values of apparent target cross section throughout the flight period. The target cross section a is further reduced to decibels of cross-sectional area relative to 1 square meter by the equation

Reentry Body
The reentry body, a 9' half-angle blunted cone, is shown in figure 4. It consisted of three main components: an ablative shield and substructure, instrumentation, and an integral 15-inch (0.381 m) spherical rocket motor. The heat shield and substructure are shown in figure 5 in a cross-section view taken across the axis of the cone. The physical properties of the reentry body are listed in table I.
8

It has been seen that the UHF radar signal was more enhanced by the ioniza- tion phenomena than the S-band radar signal. This result is reasonable to expect on the basis of the theoretical plasma and radar-frequency relationship illustrated in figures 1 and 2. It was shown that the UHF radar signal is strongly reflected from a plasma slab for a value of tenth of that required for the S-band.

The complementary effects of the shock-wave plasma and the wake plasma produce the cross-section enhancement. Two different radar frequencies are expected to have differing cross-section values from the same reentry. Because of the complexity of the reflection from and absorption in the actual plasma around and trailing the body, a sophisticated mathematical treatment is required for even approximate theoretical values for the net radar cross sections to be expected during a reentry. This treatment of the Trailblazer IIa payload is considered beyond the scope of this paper. The UHF radar cross-section value for the bare target before reentry is fairly well defined by the flight data as about 0 dB (1 square meter) this same value is approached after the reentry is complete; thus, the nose cone may have remained intact."

 

[panzerfeist1]

Well there is a conversion factor needs to be considered. Especially if you want to "translate" a unit into another. It is so weird that one need a whole long way when many simple tables and engineering books exist.

This is what i use to work with, conversion factor from book "AIAA Aerospace Design Engineers Handbook"

I have downloaded your radar calculators before with questions with regards to a sphere. 2,800mhz for S-band is 10.7cms in wavelength. After you determine the RCS for a sphere do you divide that number by the wavelength and would that determine the RCS for that frequency wavelength? There was a radar that regarded a missile in one frequency band as .002m2 RCS and the other frequency band referred it to as .6m2 RCS.

 

[Ronny]

Why is this graph saying that you are wrong? How come it says 1 meter =1m2 when we know one meter is 39 inches? Have you divided the 152o inches back to meters to confirm what you are saying is true? If you believe that the area is 1m2 = 1520 inches, than 1m2 also equals 39 meters. And no f*^(king 1m2 box would give an area of 39 meters..... That is impossible.

The graph does not say that Iam wrong, read your citation carefully.
Let say you have a square with edge length equal 1 meter. The area express in meter square is 1*1= 1 square meter. 1 meter is 39.37 inches, so the area express in square inches is 39.37*39.37 =1550 square inches.
Area of 1 square meter = area of 1550 square inches
There is nothing impossible here.

39 times 39 times 3.14 we get 4778.362426110075 inches squared. So to get this back to meters squared for a sphere we divide this number back to 39 times 39 times 3.14 to get 1m2 back. Not to add any intentional bad humor to this but you are solving the area for a sphere and after you solve the area for the sphere you think its ok to also to go solve the area of a square next and believe that its is all but one equation. 4 inches times 4 inches times 3.14 will stay as 50 inch2 sphere 1 m times 1m times 3.14 will stay as 3.14m2 sphere. 123.622 inches is in the 3.14 meter sphere, 50 inches is in the other sphere. divide the 50 inch sphere by the 123 inch sphere... To put this in layman terms you are asking to fit a sphere insided a square for your conversion. But I am fitting a 50 inch area sphere to a 3.14 meter area sphere.

If you have a circle with radius of 1 meter
The area in m2 is: 3.14.......m2
The area in inches2 is: 4869.46 inches2
We get the exact same result

We are not solving for boxes but spheres instead.

It doesn't matter. I only use square because you can't visualize sphere projection area. But the conversion factor is the same.

If thats the case convert the square inches back to meters and see if that lines up with one square meter. I am sure your answer will be no.

The answer is yes.
Check the spread sheet provided by stealthflanker.

 

[Ronny]

@Ronny

I got newer better data that pertains more to the Zircon than it does to a sphere with frequencies included in the calculation regarding RCS.
https://ntrs.nasa.gov/archive/nasa/casi.ntrs.nasa.gov/19660006803.pdf (better article)

Vehicle
The Trailblazer I1 vehicle is essentially a four-stage solid-fuel rocket system with the motors arranged in tandem. The third and fourth stages are mounted in a structural shell (velocity package) and face in a rearward direc- tion at launch. view of the velocity package, in figure 3(b). During second-stage firing, the canted fins of the rocket spin the remaining system to maintain the attitude of the velocity package at approximately 650 (up from the launch horizontal) throughout the remainder of the flight. After second-stage firing, the velocity package separates and coasts to apogee. The complete vehicle is shown in figure 3(a), and a cut-away
When the velocity package has passed apogee, the third stage fires and pro- pels the third and fourth stages downward out of the open end of the structural shell. On the Trailblazer IIa (after third-stage burnout) a l5-inch (0.381 m) spherical rocket motor mounted in the rear of the payload was fired and accel- erated the fourth stage (payload) to approximately 19,400 ft/sec (5913.1 m/sec). The trajectory of the Trailblazer has been designed to bring the reentry of the payload back toward the launch site to facilitate radar measurements. Addi- tional information on the Trailblazer IIa vehicle can be obtained from refer- ence 11 which covers the performance of several Trailblazer I1 vehicles during flight.

Before the flight, the radar are calibrated to determine the relationship between the logarithmic intermediate-frequency-amplifier voltage pulses and the apparent radar-target cross section. Signals of known power are injected into the radar receiver and the corresponding logarithmic voltages of the intermediate-frequency amplifier are recorded and plotted to establish an S-shaped signal power-voltage curve for each radar. from a standard target at given slant ranges is determined for each radar by tracking a 6-inch-diameter (0.0015 m) aluminum sphere carried aloft by a bal- loon. This information provides all the variables necessary to calculate the value of the radar constant K from the simplified standard radar equation The signal power reflected
where u is the target cross-sectional area in square meters, P, is the received power in watts, and Rr is the slant range of the target from the radar in feet (meters). in reference 13. Further information on such calibrations can be found
The radar equation must be applied to each of the 320 readings per second to develop continuous values of apparent target cross section throughout the flight period. The target cross section a is further reduced to decibels of cross-sectional area relative to 1 square meter by the equation

Reentry Body
The reentry body, a 9' half-angle blunted cone, is shown in figure 4. It consisted of three main components: an ablative shield and substructure, instrumentation, and an integral 15-inch (0.381 m) spherical rocket motor. The heat shield and substructure are shown in figure 5 in a cross-section view taken across the axis of the cone. The physical properties of the reentry body are listed in table I.
8

It has been seen that the UHF radar signal was more enhanced by the ioniza- tion phenomena than the S-band radar signal. This result is reasonable to expect on the basis of the theoretical plasma and radar-frequency relationship illustrated in figures 1 and 2. It was shown that the UHF radar signal is strongly reflected from a plasma slab for a value of tenth of that required for the S-band.

The complementary effects of the shock-wave plasma and the wake plasma produce the cross-section enhancement. Two different radar frequencies are expected to have differing cross-section values from the same reentry. Because of the complexity of the reflection from and absorption in the actual plasma around and trailing the body, a sophisticated mathematical treatment is required for even approximate theoretical values for the net radar cross sections to be expected during a reentry. This treatment of the Trailblazer IIa payload is considered beyond the scope of this paper. The UHF radar cross-section value for the bare target before reentry is fairly well defined by the flight data as about 0 dB (1 square meter) this same value is approached after the reentry is complete; thus, the nose cone may have remained intact."

View attachment 623587

I will check the details tomorrow when I have free time. But from a quick look, this study is about Trailblazer IIa and Trailblazer I1 while the reentry module in the study at the start of this topic is trailblazer Ik. So they are different target with different shape and size.
Secondly, if the bare target RCS is 0 dBsm then the result from this paper follow the same trend as what we previously see with Trailblazer Ik study

 

[panzerfeist1]

Secondly, if the bare target RCS is 0 dBsm then the result from this paper follow the same trend as what we previously see with Trailblazer Ik study

The trailblazer 2 says this in the quote, "The UHF radar cross-section value for the bare target before reentry is fairly well defined by the flight data as about 0 dB (1 square meter) this same value is approached after the reentry is complete; thus, the nose cone may have remained intact." They show the image on the upper right hand corner with what is seen as a nose cone. and they have drawn the conclusion that the RCS of 1m2 before and after re-enty was the same, because the cone was still attached.



The graph I have presented is what the radar sees of the cone which depends on the aspect angle of the target. I will have to find a calculator to see what happens when a target cruises at 40kms constantly, than what a ground radar would see of that approaching target. I will be done for today until than.